Common Pole–Polar Properties of Central Catadioptric Sphere and Line Images Used for Camera Calibration

Abstract

Central catadioptric cameras with a single effective viewpoint contain both mirrors and pinhole cameras that increase the imaging field of view. In this study, the common pole–polar properties of central catadioptric sphere or line images are investigated and used for camera calibration. From these properties, the pole and polar with respect to the image of absolute conic and the modified image of absolute conic, respectively, can be recovered according to the generalized eigenvalue decomposition. Moreover, these techniques are valid for paracatadioptric sensors with the degenerate conic dual to the circular points being considered. At least three images of spheres or lines are required to completely calibrate any central catadioptric camera. The intrinsic parameters of the camera, the shape of reflective mirror, and the distortion parameters can be linearly estimated using the algebraic and geometric constraints of the sphere or line images obtained by the central catadioptric camera. The obtained experimental results demonstrate the effectiveness and feasibility of the proposed calibration algorithm.

Appendices

Appendix A

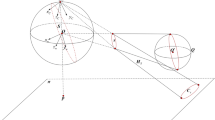
In the MCS, the unit normal vectors of planes ${{\varvec{\pi }} _m}$ and ${{\varvec{\pi } _S}}$ are ${{\varvec{O}}_c}{\varvec{O}}\mathrm{{ = }}{\left[ {\begin{array}{*{20}{c}} 0&0&1 \end{array}} \right] ^T}$ and ${{\varvec{O}}_c}{\varvec{N}}\mathrm{{ = }}{\left[ {\begin{array}{*{20}{c}} {{n_x}}&{{n_y}}&{{n_z}} \end{array}} \right] ^T}$, respectively. Since the line ${{\varvec{O}}_c}{\varvec{O}}$ and the normal ${{\varvec{O}}_c}{\varvec{N}}$ are coplanar, the corresponding projection points $\bar{{\varvec{O}}}\mathrm{{ = }}{\left[ {\begin{array}{*{20}{c}} 0&0&1 \end{array}} \right] ^T}$ and $\bar{{\varvec{N}}}\mathrm{{ = }}{\left[ {\begin{array}{*{20}{c}} {{n_x}}&{{n_y}}&{{n_z}} \end{array}} \right] ^T}$ are collinear. Further, the intersection line ${\varvec{D}}$ of planes ${\varvec{\pi } _m}$ and ${\varvec{\pi } _S}$ is orthogonal to the plane containing both the normal ${{\varvec{O}}_c}{\varvec{N}}$ and ${{\varvec{O}}_c}{\varvec{O}}$. Considering two $3 \times 3$ symmetric matrices ${\bar{{\varvec{C}}}_m}$ and ${\bar{{\varvec{C}}}}$, and solving Eq. (9) yields the generalized eigenvalue ${\lambda _1}$ of $\left( {{{\bar{{\varvec{C}}}}_m},\bar{{\varvec{C}}}} \right)$ and the corresponding generalized eigenvector ${\bar{{\varvec{v}}}_1}$ (computed by MAPLE) as ${\lambda _1} = {\left( {{d_0} - \xi {n_z}} \right) ^2}$ and ${\bar{{\varvec{v}}}_1} = {\left[ {\begin{array}{*{20}{c}} { - {n_y}}&{{n_x}}&0 \end{array}} \right] ^T}$, respectively. Thus, the point ${\bar{{\varvec{v}}}_1} = {\left[ {\begin{array}{*{20}{c}} { - {n_y}}&{{n_x}}&0 \end{array}} \right] ^T}$ is an infinity point of the line ${\varvec{D}}$. In Fig. 2, according to Eq. (9), the common polar corresponding to ${\bar{{\varvec{v}}}_1}$ with respect to ${\bar{{\varvec{C}}}_m}$ and ${\bar{{\varvec{C}}}}$ aligns with the major axis $\bar{\varvec{\mu }} = {\bar{\varvec{\mu }} _1} = {\left[ {\begin{array}{*{20}{c}} { - {n_y}}&{{n_x}}&0 \end{array}} \right] ^T}$ (see Table 1) formed by $\bar{{\varvec{O}}}$ and $\bar{{\varvec{N}}}$ Barreto and Araujo (2005). Hence, according to the Definition 4, the infinite point ${\bar{{\varvec{v}}} _1}$ and the line ${\bar{\varvec{\mu }} _1}$ also exhibit a pole–polar relationship with respect to the absolute conic ${\bar{\varvec{\Omega }} _\infty }$(not depicted) Andrew (2001). As the projectivity ${{\varvec{H}}_c}$ preserves incidence and collinearity, the property holds in the central catadioptric image plane after the transformation ${{\varvec{H}}_c}$. Thus, the pole–polar relation between ${\hat{{\varvec{v}}}_1}$ and ${\hat{\varvec{\mu }} _1}$, which corresponds to the common pole and polar for ${\hat{{\varvec{C}}}_m}$ and ${\hat{{\varvec{C}}}}$, respectively, is preserved under the projection transformation ${{\varvec{H}}_c}$. Notably, the locus ${\hat{\varvec{\mu }} _1}$ is no longer the major axis of the catadioptric sphere or the line image ${\hat{{\varvec{C}}}}$. However, from Fig. 2, the point ${\hat{{\varvec{v}}}_1}$ and line ${\hat{\varvec{\mu }} _1}$ can be uniquely determined from the generalized eigenvectors of the conics ${\hat{{\varvec{C}}}_m}$ and ${\hat{{\varvec{C}}}}$ , as ${\hat{\varvec{\mu }} _1}$ is the only line intersecting both ${\hat{{\varvec{C}}}_m}$ and ${\hat{{\varvec{C}}}}$ at two points.

Appendix B

For a paracatadioptric camera, according to Eq. (4), replacing $\xi$ by 1 yields

$$\begin{aligned} {{\bar{{\varvec{C}}}_S} = \left[ {\begin{array}{*{20}{c}} {{{({d_0} - {m_z})}^2}}&{}\mathrm{{0}}&{}{({d_0} - {m_z}){m_x}}\\ \mathrm{{0}}&{}{{{({d_0} - {m_z})}^2}}&{}{({d_0} - {m_z}){m_y}}\\ {({d_0} - {m_z}){m_x}}&{}{({d_0} - {m_z}){m_y}}&{}{({d_0}^2 - {m_z}^2)} \end{array}} \right] } \end{aligned}$$

(B1)

Consider the dual of circle ${\bar{{\varvec{C}}}_S}^\mathrm{{*}}$, which satisfies

$$\begin{aligned} {{\bar{{\varvec{C}}}_S}^\mathrm{{*}} = \left[ {\begin{array}{*{20}{c}} {{d_0}^2 + {m_x}^2 - 1}&{}{{m_x}{m_y}}&{}{({d_0} - {m_z}){m_x}}\\ {{m_x}{m_y}}&{}{{d_0}^2 + {m_x}^2 - 1}&{}{({d_0} - {m_z}){m_y}}\\ {({d_0} - {m_z}){m_x}}&{}{({d_0} - {m_z}){m_y}}&{}{{{({d_0} - {m_z})}^\mathrm{{2}}}} \end{array}} \right] }\nonumber \\ \end{aligned}$$

(B2)

From Eq. (7), the dual of the conic curve ${\hat{{\varvec{C}}}^ * }$ is the paracatadioptric image of a line or sphere in the scene satisfying

$$\begin{aligned} {{\lambda _w}^*{\hat{{\varvec{C}}}^*} = {{\varvec{H}}_c}{\bar{{\varvec{C}}}_S}^\mathrm{{*}}{{\varvec{H}}_c}^T} \end{aligned}$$

(B3)

By simplification, equation Eq. B3 can be rearranged as

$$\begin{aligned} {{\lambda _w}^*{\hat{{\varvec{C}}}^*} = {\hat{{\varvec{C}}}_\infty ^*}+{\hat{{\varvec{v}}}}^*{\hat{{\varvec{v}}}}^*}^T \end{aligned}$$

(B4)

where ${\hat{{\varvec{C}}}_\infty }^*\mathrm{{ = }}{{\varvec{H}}_c}\left[ {\begin{array}{*{20}{c}} \mathrm{{1}}&{}\mathrm{{0}}&{}\mathrm{{0}}\\ \mathrm{{0}}&{}\mathrm{{1}}&{}\mathrm{{0}}\\ \mathrm{{0}}&{}\mathrm{{0}}&{}\mathrm{{0}} \end{array}} \right] {{\varvec{H}}_c}^T$ is the projection of the CDCP, ${\hat{{\varvec{v}}}^*} = \frac{1}{{\sqrt{\left( {1 - {d_0}^2} \right) } }}{{\varvec{H}}_c}\left[ {\begin{array}{*{20}{c}} {{m_x}}\\ {{m_y}}\\ {{d_0} - {m_z}} \end{array}} \right]$. In a similar manner, from Proposition 4, ${\hat{{\varvec{C}}}_\infty }^*$ can be computed by considering the generalized eigenvectors of any two line or sphere images. Note that, from Eq. B4 , for paracatadioptric systems, the dual of the line or sphere image is in double contact with a degenerated-line (envelope) conic $\hat{{\varvec{C}}}_\infty ^*$ consisting of the image of the circular points. Similar results can be obtained using the method presented by Ying and Zha (2008).